 |
 |
|
 |
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
Mostly because they didn't have a picture to go with the Wikipedia article...
https://en.wikipedia.org/wiki/Curve_of_constant_width
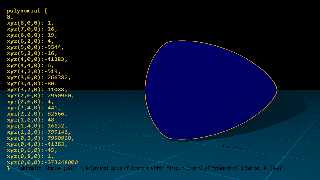
So here it is folks, the eighth-order polynomial defined by:
(x^2+y^2)^4 - 45(x^2+y^2)^3 - 41283(x^2+y^2)^2 + 7950960(x^2+y^2) +
16(x^2-3y^2)^3 + 48(x^2+y^2)(x^2-3y^2)^2 + (x^2-3y^2)x[16(x^2+y^2)^2 -
5544(x^2+y^2)+266382] - 720^3
[also see https://arxiv.org/pdf/1504.06733.pdf pg. 21]
Post a reply to this message
Attachments:
Download 'polynomial1.png' (316 KB)
Preview of image 'polynomial1.png'

|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
> Mostly because they didn't have a picture to go with the Wikipedia article...
>
> https://en.wikipedia.org/wiki/Curve_of_constant_width
>
>
> So here it is folks, the eighth-order polynomial defined by:
>
> (x^2+y^2)^4 - 45(x^2+y^2)^3 - 41283(x^2+y^2)^2 + 7950960(x^2+y^2) +
> 16(x^2-3y^2)^3 + 48(x^2+y^2)(x^2-3y^2)^2 + (x^2-3y^2)x[16(x^2+y^2)^2 -
> 5544(x^2+y^2)+266382] - 720^3
>
>
> [also see https://arxiv.org/pdf/1504.06733.pdf pg. 21]
>
And you did not already added it to the wiki page ?
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
Alain <kua### [at] videotron ca> wrote:
> > Mostly because they didn't have a picture to go with the Wikipedia article...
> And you did not already added it to the wiki page ?
Nope:
1. I didn't have a wikipedia account
[I do now]
2. When I tried to edit the article, some automated filter claimed the edit
didn't add anything important - or something similar.
I'll give it a another go when I have some more free time and I better
understand how to edit a wiki page. ca> wrote:
> > Mostly because they didn't have a picture to go with the Wikipedia article...
> And you did not already added it to the wiki page ?
Nope:
1. I didn't have a wikipedia account
[I do now]
2. When I tried to edit the article, some automated filter claimed the edit
didn't add anything important - or something similar.
I'll give it a another go when I have some more free time and I better
understand how to edit a wiki page.
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
"Bald Eagle" <cre### [at] netscape net> wrote:
>
> I'll give it a another go when I have some more free time and I better
> understand how to edit a wiki page.
Good! Then you can teach me how to do it ;-) net> wrote:
>
> I'll give it a another go when I have some more free time and I better
> understand how to edit a wiki page.
Good! Then you can teach me how to do it ;-)
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
Am 10/21/2017 um 3:51 schrieb Bald Eagle:
> Mostly because they didn't have a picture to go with the Wikipedia article...
>
> https://en.wikipedia.org/wiki/Curve_of_constant_width
>
>
> So here it is folks, the eighth-order polynomial defined by:
>
> (x^2+y^2)^4 - 45(x^2+y^2)^3 - 41283(x^2+y^2)^2 + 7950960(x^2+y^2) +
> 16(x^2-3y^2)^3 + 48(x^2+y^2)(x^2-3y^2)^2 + (x^2-3y^2)x[16(x^2+y^2)^2 -
> 5544(x^2+y^2)+266382] - 720^3
>
>
> [also see https://arxiv.org/pdf/1504.06733.pdf pg. 21]
>
Please do not use this 3d-look text (that even throws shadows) with this
image. Besides that it IMHO does not look good it is more importantly
very hard to read.
And personally - e.g. on a wiki-page - I hate text information where
copy and paste doesn't work ;)
-Ive
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
On 25/10/2017 09:29, Ive wrote:
> Am 10/21/2017 um 3:51 schrieb Bald Eagle:
>> Mostly because they didn't have a picture to go with the Wikipedia
>> article...
>>
>> https://en.wikipedia.org/wiki/Curve_of_constant_width
>>
>>
>> So here it is folks, the eighth-order polynomial defined by:
>>
>> (x^2+y^2)^4 - 45(x^2+y^2)^3 - 41283(x^2+y^2)^2 + 7950960(x^2+y^2) +
>> 16(x^2-3y^2)^3 + 48(x^2+y^2)(x^2-3y^2)^2 + (x^2-3y^2)x[16(x^2+y^2)^2 -
>> 5544(x^2+y^2)+266382] - 720^3
>>
>>
>> [also see https://arxiv.org/pdf/1504.06733.pdf pg. 21]
>>
>
> Please do not use this 3d-look text (that even throws shadows) with this
> image. Besides that it IMHO does not look good it is more importantly
> very hard to read.
Oh! I liked it.
> And personally - e.g. on a wiki-page - I hate text information where
> copy and paste doesn't work ;)
>
In that case you might like capture2text. It is a utility that takes a
screenshot of part of your screen then OCR's it and puts the text in the
clipboard. I've been using it for a couple of years.
Although it doesn't work too well with Bald Eagle's 3D text.
It can also use Google Translate.
http://capture2text.sourceforge.net/
--
Regards
Stephen
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
On 25-10-2017 11:29, Stephen wrote:
> On 25/10/2017 09:29, Ive wrote:
>> Am 10/21/2017 um 3:51 schrieb Bald Eagle:
>>> Mostly because they didn't have a picture to go with the Wikipedia
>>> article...
>>>
>>> https://en.wikipedia.org/wiki/Curve_of_constant_width
>>>
>>>
>>> So here it is folks, the eighth-order polynomial defined by:
>>>
>>> (x^2+y^2)^4 - 45(x^2+y^2)^3 - 41283(x^2+y^2)^2 + 7950960(x^2+y^2) +
>>> 16(x^2-3y^2)^3 + 48(x^2+y^2)(x^2-3y^2)^2 + (x^2-3y^2)x[16(x^2+y^2)^2 -
>>> 5544(x^2+y^2)+266382] - 720^3
>>>
>>>
>>> [also see https://arxiv.org/pdf/1504.06733.pdf pg. 21]
>>>
>>
>> Please do not use this 3d-look text (that even throws shadows) with
>> this image. Besides that it IMHO does not look good it is more
>> importantly very hard to read.
>
> Oh! I liked it.
Me too, but I must agree with Ive here. I would also add (sorry for
this) that the object texture is misleading: it looks like a 3D surface,
curved towards the viewer, while it is flat in reality. It took me a
while before I understood what I was looking at.
--
Thomas
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
Ive <ive### [at] lilysoft org> wrote:
> Please do not use this 3d-look text (that even throws shadows) with this
> image. Besides that it IMHO does not look good it is more importantly
> very hard to read.
I usually use pretty flat, functional text, and I did think about the look and
readability. I didn't think it looked TOO bad, but perhaps for a wikipedia
page, it out to be a little less artistic.
> And personally - e.g. on a wiki-page - I hate text information where
> copy and paste doesn't work ;)
Perhaps I can add the text info as plain text or formatted text or MathJax, but
I wanted to have the info in the graphic too - for inseparability purposes.
Along those lines, I wouldn't mind embedding the full POV-Ray scene used to
generate the graphic in the metadata header section of the file - I just have to
puzzle out _exactly_ how.
Thanks for the constructive feedback!
> -Ive org> wrote:
> Please do not use this 3d-look text (that even throws shadows) with this
> image. Besides that it IMHO does not look good it is more importantly
> very hard to read.
I usually use pretty flat, functional text, and I did think about the look and
readability. I didn't think it looked TOO bad, but perhaps for a wikipedia
page, it out to be a little less artistic.
> And personally - e.g. on a wiki-page - I hate text information where
> copy and paste doesn't work ;)
Perhaps I can add the text info as plain text or formatted text or MathJax, but
I wanted to have the info in the graphic too - for inseparability purposes.
Along those lines, I wouldn't mind embedding the full POV-Ray scene used to
generate the graphic in the metadata header section of the file - I just have to
puzzle out _exactly_ how.
Thanks for the constructive feedback!
> -Ive
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
Thomas de Groot <tho### [at] degroot org> wrote:
> Me too, but I must agree with Ive here. I would also add (sorry for
> this) that the object texture is misleading: it looks like a 3D surface,
> curved towards the viewer, while it is flat in reality. It took me a
> while before I understood what I was looking at.
>
> --
> Thomas
It's not really textured - I have it lit with 4 lights - white, magenta, yellow,
and blue.
It IS 3D - just not concave or convex, since the z components are all zero, and
that winds up giving a polynomial object that is infinitely scaled in the
z-direction. So I intersected it with a thin box.
But I take your meaning.
This leads into a topic about the Documentation that I will post in a fresh
thread.
No need for apologies: it's constructive, and honest feedback about how the
render comes out. org> wrote:
> Me too, but I must agree with Ive here. I would also add (sorry for
> this) that the object texture is misleading: it looks like a 3D surface,
> curved towards the viewer, while it is flat in reality. It took me a
> while before I understood what I was looking at.
>
> --
> Thomas
It's not really textured - I have it lit with 4 lights - white, magenta, yellow,
and blue.
It IS 3D - just not concave or convex, since the z components are all zero, and
that winds up giving a polynomial object that is infinitely scaled in the
z-direction. So I intersected it with a thin box.
But I take your meaning.
This leads into a topic about the Documentation that I will post in a fresh
thread.
No need for apologies: it's constructive, and honest feedback about how the
render comes out.
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
Simpler version
Post a reply to this message
Attachments:
Download 'polynomial1.png' (224 KB)
Preview of image 'polynomial1.png'
|
 |
|  |
|  |
|
 |
|
 |
|  |




![]()