I try to download moray, but failed.

http://www.stmuc.com/moray/

The requested URL was not found on this server.

Are there other ways to download it?

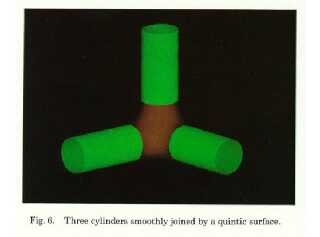
I want to implement some algebraic blending algorithm to moothly join two or
more algebraic surfaces.

Post a reply to this message

Attachments:
Download 'blending.jpg' (40 KB)

Preview of image 'blending.jpg'

"ghzhao" <ghz### [at] dluteducn> wrote:
> I try to download moray, but failed.
>
> http://www.stmuc.com/moray/
>
> The requested URL was not found on this server.
>
> Are there other ways to download it?
>
> I want to implement some algebraic blending algorithm to moothly join two or
> more algebraic surfaces.

You can use the Wayback machine:

http://web.archive.org/web/20220331032107/http://www.stmuc.com/moray/medown.html

Also, if you model those surfaces as isosurfaces, you can easily blend them
together.
https://news.povray.org/povray.binaries.images/thread/%3Cweb.5d4b7ce3a683fa3a4eec112d0%40news.povray.org%3E/

Or you can possibly figure out where to position them so that the surface
tangents are equal, and you gt a smooth, continuous curve.

http://news.povray.org/povray.binaries.images/thread/%3Cweb.5da27a2a65c96eb4eec112d0%40news.povray.org%3E/?ttop=441843&
toff=500

- BW

Post a reply to this message

hi,

"ghzhao" <ghz### [at] dluteducn> wrote:
> I try to download moray, but failed.
> ...
> I want to implement some algebraic blending algorithm to moothly join two or
> more algebraic surfaces.

out of interest (although I haven't the maths), is there a reason you cannot (or
do not want to) use POV-Ray's own SDL to implement the "blending" algorithm ?


regards, jr.

Post a reply to this message