Bill Pragnell wrote:
> Nice image, all the nicer for the colour bleeding!

Thanks!

> Surely the Miller indices define the crystal planes, 

Yes....

> so you can use them
> directly to define your intersection planes (i.e., no knowledge of angles
> required)?

No. I wish that were true, because it would make everything much easier. 
Now, it *might* be true for cubic crystal systems, but I have to check 
on that. For hexagonal systems that theory just doesn't hold.

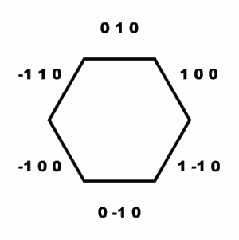
Look at the attached image. If we were talking just straight normals 
here, the numbers would be decimals or very large integers. Instead, we 
have angles represented by three small integers, ranging between -1 and 
1 (they can be higher, I've seen them as high as 6).

I don't understand how the Miller indices work, but I know it has to do 
with how molecules in the crystal are offset from one another. The 
Wikipedia article just confused me, as I have no formal training in 
higher mathematics.

If you have a way to calculate the normals for single faces (not the 
angle between two faces), by all means, let me know! Otherwise, I'm off 
to try the answer via Google....

Sam

Post a reply to this message

Attachments:
Download 'miller_indices.gif' (3 KB)

Preview of image 'miller_indices.gif'