 |
 |
|
 |
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
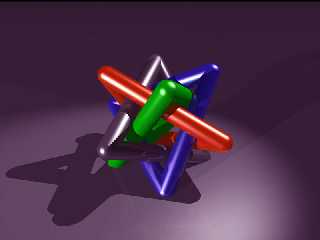
While morphing between a snubcube and a cuboctahedron
I spotted a nice arrangement of diagonals forming these
interlocking triangles.
Jim Buddenhagen
Post a reply to this message
Attachments:
Download '4tris_cyl_test_800.jpg' (35 KB)
Preview of image '4tris_cyl_test_800.jpg'
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
James Buddenhagen wrote:
> While morphing between a snubcube and a cuboctahedron
> I spotted a nice arrangement of diagonals forming these
> interlocking triangles.
>
> Jim Buddenhagen
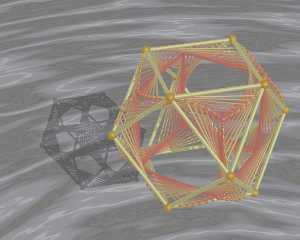
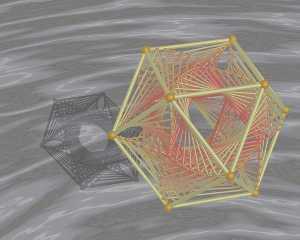
That is very nice! I was playing around with the same shapes (cube and
octahedron), a while ago, but never noticed the diagonal vertices. What
I did notice, was that rotating the faces of the cube 45 degrees and
out, gives the same shape as rotating the faces of the octahedron 60
degrees and out -- and the vertices are identically those of your
interlocking triangles (what is it, a snubcube or a cuboctahedron?) But
the triangles -- that's really fascinating.
Dave Matthews
Post a reply to this message
Attachments:
Download 'crsn_01.jpg' (38 KB)
Download 'orsn_01.jpg' (41 KB)
Preview of image 'crsn_01.jpg'
Preview of image 'orsn_01.jpg'
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
"Dave Matthews" <n@nn.n> wrote in message news:4212d2f4@news.povray.org...
> James Buddenhagen wrote:
> > While morphing between a snubcube and a cuboctahedron
> > I spotted a nice arrangement of diagonals forming these
> > interlocking triangles.
> >
> > Jim Buddenhagen
> That is very nice! I was playing around with the same shapes (cube and
> octahedron), a while ago, but never noticed the diagonal vertices. What
> I did notice, was that rotating the faces of the cube 45 degrees and
> out, gives the same shape as rotating the faces of the octahedron 60
> degrees and out -- and the vertices are identically those of your
> interlocking triangles (what is it, a snubcube or a cuboctahedron?) But
> the triangles -- that's really fascinating.
>
> Dave Matthews
Interesting pictures, but I'm not sure I see the same vertices. In any case the
vertices of 'my' three triangles were vertices of the cubeoctahedron, namely:
triangle 1: <-1, 1, 0>, <0, -1, 1>, <1, 0, -1>
triangle 2: <-1, -1, 0>, <0, 1, -1>, <1, 0, 1>
triangle 3: <-1, 0, 1>, <0, -1, -1>, <1, 1, 0>
triangle 4: <0, 1, 1>, <1, -1, 0>, <-1, 0, -1>
I put a crude wireframe animation of the morphing of cubeoctahedron to snubcube
temporarily here: http://www.buddenbooks.com/jb/misc/cubeoct_snubcube_anim.gif
(306k)
Jim Buddenhagen
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
"James Buddenhagen" <jbu### [at] REMOVEtexas net> wrote:
> Interesting pictures, but I'm not sure I see the same vertices. In any case the
> vertices of 'my' three triangles were vertices of the cubeoctahedron, namely:
>
> triangle 1: <-1, 1, 0>, <0, -1, 1>, <1, 0, -1>
> triangle 2: <-1, -1, 0>, <0, 1, -1>, <1, 0, 1>
> triangle 3: <-1, 0, 1>, <0, -1, -1>, <1, 1, 0>
> triangle 4: <0, 1, 1>, <1, -1, 0>, <-1, 0, -1>
>
> I put a crude wireframe animation of the morphing of cubeoctahedron to snubcube
> temporarily here: http://www.buddenbooks.com/jb/misc/cubeoct_snubcube_anim.gif
> (306k)
>
> Jim Buddenhagen
The gold balls on my pictures (the vertices of the yellow wireframe shapes)
form the vertices of your cubeoctahedron. The 4 at the top correspond to
the 4 vertices at the top of your 4 intersecting triangles, etc. In fact,
the yellow frames in my pictures look identical to the start of your .gif
To see my "morphing" in reverse, take your initial gif cubeoctahedron, look
at the square faces, rotate each of them by 45 degrees and collapse them
in, until they fit snug -- you get a cube. Now take the triangular faces,
rotate them each by 60 degrees and collapse them in -- you get an
octahedron.
Anyway, I noticed the cubeoctahedron shape of the vertices of your triangle,
and recognized the resulting shape of my outward twists of cube and
octahedron (which would make sense.)
Your morphing .gif is interesting, also. Thanks.
Dave Matthews net> wrote:
> Interesting pictures, but I'm not sure I see the same vertices. In any case the
> vertices of 'my' three triangles were vertices of the cubeoctahedron, namely:
>
> triangle 1: <-1, 1, 0>, <0, -1, 1>, <1, 0, -1>
> triangle 2: <-1, -1, 0>, <0, 1, -1>, <1, 0, 1>
> triangle 3: <-1, 0, 1>, <0, -1, -1>, <1, 1, 0>
> triangle 4: <0, 1, 1>, <1, -1, 0>, <-1, 0, -1>
>
> I put a crude wireframe animation of the morphing of cubeoctahedron to snubcube
> temporarily here: http://www.buddenbooks.com/jb/misc/cubeoct_snubcube_anim.gif
> (306k)
>
> Jim Buddenhagen
The gold balls on my pictures (the vertices of the yellow wireframe shapes)
form the vertices of your cubeoctahedron. The 4 at the top correspond to
the 4 vertices at the top of your 4 intersecting triangles, etc. In fact,
the yellow frames in my pictures look identical to the start of your .gif
To see my "morphing" in reverse, take your initial gif cubeoctahedron, look
at the square faces, rotate each of them by 45 degrees and collapse them
in, until they fit snug -- you get a cube. Now take the triangular faces,
rotate them each by 60 degrees and collapse them in -- you get an
octahedron.
Anyway, I noticed the cubeoctahedron shape of the vertices of your triangle,
and recognized the resulting shape of my outward twists of cube and
octahedron (which would make sense.)
Your morphing .gif is interesting, also. Thanks.
Dave Matthews
Post a reply to this message
|
 |
|  |
|  |
|
 |
|
 |
|  |
|  |
|
 |
"Dave Matthews" <dma### [at] wr mnwest mnwest mnscu mnscu edu> wrote in message
news:web.42139c1c7cff8ff58c7259570@news.povray.org...
> "James Buddenhagen" <jbu### [at] REMOVEtexas edu> wrote in message
news:web.42139c1c7cff8ff58c7259570@news.povray.org...
> "James Buddenhagen" <jbu### [at] REMOVEtexas net> wrote:
> > Interesting pictures, but I'm not sure I see the same vertices. In any case
the
> > vertices of 'my' three triangles were vertices of the cubeoctahedron,
namely:
> >
> > triangle 1: <-1, 1, 0>, <0, -1, 1>, <1, 0, -1>
> > triangle 2: <-1, -1, 0>, <0, 1, -1>, <1, 0, 1>
> > triangle 3: <-1, 0, 1>, <0, -1, -1>, <1, 1, 0>
> > triangle 4: <0, 1, 1>, <1, -1, 0>, <-1, 0, -1>
> >
> > I put a crude wireframe animation of the morphing of cubeoctahedron to
snubcube
> > temporarily here:
http://www.buddenbooks.com/jb/misc/cubeoct_snubcube_anim.gif
> > (306k)
> >
> > Jim Buddenhagen
>
> The gold balls on my pictures (the vertices of the yellow wireframe shapes)
> form the vertices of your cubeoctahedron. The 4 at the top correspond to
> the 4 vertices at the top of your 4 intersecting triangles, etc. In fact,
> the yellow frames in my pictures look identical to the start of your .gif
>
> To see my "morphing" in reverse, take your initial gif cubeoctahedron, look
> at the square faces, rotate each of them by 45 degrees and collapse them
> in, until they fit snug -- you get a cube. Now take the triangular faces,
> rotate them each by 60 degrees and collapse them in -- you get an
> octahedron.
>
> Anyway, I noticed the cubeoctahedron shape of the vertices of your triangle,
> and recognized the resulting shape of my outward twists of cube and
> octahedron (which would make sense.)
>
> Your morphing .gif is interesting, also. Thanks.
>
> Dave Matthews
Here is a pic that shows how 'my' triangles fit into a cubeoctahedron.
The 4 vertices at the top, as you say, are the top vertices of
the 4 triangles.
Jim Buddenhagen net> wrote:
> > Interesting pictures, but I'm not sure I see the same vertices. In any case
the
> > vertices of 'my' three triangles were vertices of the cubeoctahedron,
namely:
> >
> > triangle 1: <-1, 1, 0>, <0, -1, 1>, <1, 0, -1>
> > triangle 2: <-1, -1, 0>, <0, 1, -1>, <1, 0, 1>
> > triangle 3: <-1, 0, 1>, <0, -1, -1>, <1, 1, 0>
> > triangle 4: <0, 1, 1>, <1, -1, 0>, <-1, 0, -1>
> >
> > I put a crude wireframe animation of the morphing of cubeoctahedron to
snubcube
> > temporarily here:
http://www.buddenbooks.com/jb/misc/cubeoct_snubcube_anim.gif
> > (306k)
> >
> > Jim Buddenhagen
>
> The gold balls on my pictures (the vertices of the yellow wireframe shapes)
> form the vertices of your cubeoctahedron. The 4 at the top correspond to
> the 4 vertices at the top of your 4 intersecting triangles, etc. In fact,
> the yellow frames in my pictures look identical to the start of your .gif
>
> To see my "morphing" in reverse, take your initial gif cubeoctahedron, look
> at the square faces, rotate each of them by 45 degrees and collapse them
> in, until they fit snug -- you get a cube. Now take the triangular faces,
> rotate them each by 60 degrees and collapse them in -- you get an
> octahedron.
>
> Anyway, I noticed the cubeoctahedron shape of the vertices of your triangle,
> and recognized the resulting shape of my outward twists of cube and
> octahedron (which would make sense.)
>
> Your morphing .gif is interesting, also. Thanks.
>
> Dave Matthews
Here is a pic that shows how 'my' triangles fit into a cubeoctahedron.
The 4 vertices at the top, as you say, are the top vertices of
the 4 triangles.
Jim Buddenhagen
Post a reply to this message
Attachments:
Download '4tris_cyl_test2.jpg' (37 KB)
Preview of image '4tris_cyl_test2.jpg'

|
 |
|  |
|  |
|
 |
|
 |
|  |
|
 |




![]()